-
Null space and column space basisVector and Space 2021. 6. 23. 15:44
What I want to share in this blog is a basis of null space and column space.
1. a null space and a column space: I told you these in an earlier blog, but I'm going to talk about these again for a quick review. the null space is a set that contains vectors that make an equation a matrix A times a vector x 0. Here it is.
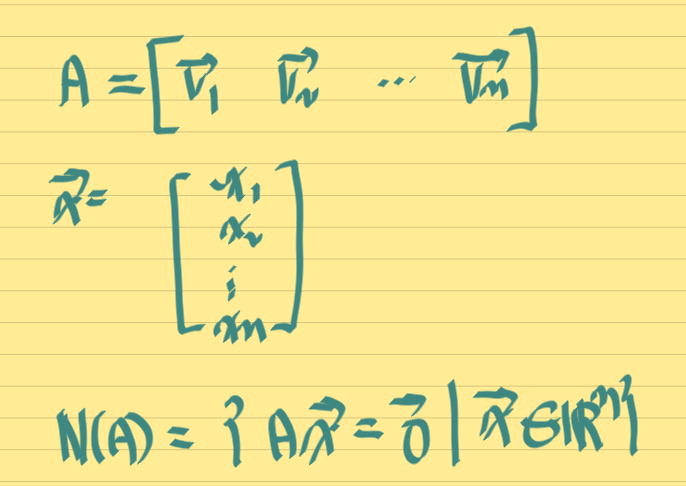
column space is a lot easier to understand, which is a set of column vectors in matrix A.

** Note that if the null space of A only contains the 0 vector, then these vectors are linearly independent.
2. A basis: the basis is a set of vectors that span a subspace, and they are also linearly independent. In order to make a column space linearly independent, then you have to remove all the free variables in the vector set.
'Vector and Space' 카테고리의 다른 글
Column space of a matrix (0) 2021.06.21 Null space : Linear Independence (0) 2021.06.03 Null space of a matrix introduction (0) 2021.05.31 The multiplication of a matrix vector (0) 2021.05.31 Understanding of a function (0) 2021.01.01